0570 Maths P1 - June 2024
1. Given that 36 ÷ 9 = 4, 4 is known as the
A. Remainder
B. Divisor
C. Quotient
D. Dividend
2. The next prime number greater than 23 is
3. The absolute value of - 1 - 9 is
4. Converting 0.15 to a fraction in its simplest form gives
\[\text{B.} \frac{\text{3}}{\text{20}}\]
\[\text{B.} \frac{\text{15}}{\text{100}}\]
\[\text{C.} \frac{\text{3}}{\text{100}}\]
\[\text{D.} \frac{\text{15}}{\text{20}}\]
5. Expressing 0.0597 in standard for gives
A. 5.97 x `10^2`
B. 5.97 x `10^-2`
C. 6.0 x `10^-2`
D. 6.0 x `10^2`
6. The number of significant figures in 14.501 is
7. Mary Went to bed at 11:20 pm and asked John to wake her up after 2 hours. The time by the clock that John woke up Mary is
A. 13:20 pm
B. 1:20 pm
C. 1:20
D. 13:20
8. An article is sold at a loss of 10%. Given that the selling price is 7,200 FCFA. The cost price in FCFA is
A. 7,920
B. 6,480
C. 8,800
D. 8,000
9. Given that six textbook each of the same price cost 30,000 FCFA. The cost in FCFA of three textbooks is
A. 5,000
B. 15,000
C. 90,000
D. 25,000
10. The scale of a map is 1:50,000. The actual length in km of a raod measuring 4cm on the map is
11. Given the sets A and B Such that A = {1, 2, 3, 4} and B = {2, 4, 6, 8}. The sets A and B Are called
A. Equal sets
B. Power sets
C. Equivalent sets
D. Subsets
12. Given that P and Q are disjoint sets. The relationship between P and Q in set notation is
A. P U Q = Ø
B. n(P ∩ Q) = 0
C. P n Q = (Ø)
D. P = Q
13.The shaded region in figure 1 is represented as
A. A U B
B. (A ∩ B)'
C. (A U B)'
D. A' U B'
14. The negation of "Some students will pass the examination" is
A. No student will pass the examination
B. No student will not pass the examination
C. All students will not pass the examination
D. All students will pass the examination
15. Figure 2 is an arrow diagram of a relation between P and Q. The range of the relation is
A. {2, 5}
B. {1, 2, 3, 4, 5}
C. {a, b, c}
D. {1, 3, 4}
16. Given the function f(x) = 1 - x. The value of f(-3) is
17. A and B are two sets such that A = {3, 4} and B = {x, y} , the cartesian product A X B is
A. {(3, x), (4, y)}
B. (3x, 4y)
C. {(3, x), (3, y), (4, x), (4, y)}
D. (3,x), (3,y), (4,x), (4, y)
18. The function f is defined on R, the set of real numbers by f:x --> = 3x - 2, `f^-1`:x -->
\[\text{A.} \frac{\text{x + 2}}{\text{3}}\]
\[\text{A.} \frac{\text{x - 2}}{\text{3}}\]
\[\text{A.} \frac{\text{x + 3}}{\text{2}}\]
\[\text{A.} \frac{\text{x - 3}}{\text{2}}\]
19. A polygon having nine sides is called
A. Octagon
B. Decagon
C. Nonagon
D. Heptagon
20. The sum of the three interior angles of a triangle is
A. 90°
B. 270°
C. 360°
D. 180°
21. The number of lines of symmetry in rhombus is/are
22. Figure 3 is a circle with center O and a chord PQ subtending an angle of 70° at the center, O. The value of the angle marked θ is
A. 35°
B. 70°
C. 55°
D. 20°
23. Figure 4 represents the net of
A. a rectangle
B. a cylinder
C. a square
D. an open cylinder
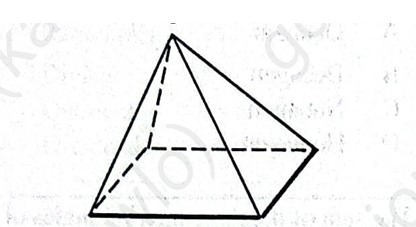
24. Figure 5 is rectangular based pyramid. The number of edges is:
25. Figure 6 is a circle with center O. The shaded region in the figure is called a
A. Segment
B. Sector
C. Chord
D. Secant
26. The perimeter of a rectangle with length x units and width y units in terms of x and y is
A. 2xy
B. x + y
C. xy
D. 2(x + y)
27. Figure 7 is a right-angled triangle with sides 6cm, x cm and area `24cm^2`. The value of x in the figure is
28. The volume in cm3 of a cone with height 10cm and base radius 6cm in terms of π is
A. 60 π
B. 360 π
C. 120 π
D. 180 π
29. The y-intercept of the line y = 2x + 4 on the cartesian plane is
30. The gradient of the line 3y = 6x - 3
31. tHE equation of the line \(l\) in the cartesian plane shown in figure 8 is
A. y = 4
B. x = 4
C. y = 4x
D. 4y = x
32. Subtracting 2 + b from 2a + b gives
A. 2 - 2a
B. 2a + 2
C. 2a - 2
D. 2a + 2b - 2
33. The value of the expression `x^2 - y` when x = 3 and y = 2 is
34. The range of values of x for which 7 - 2x ≤ 19 is
A. x ≥ -6
B. x ≤ -6
C. x ≤ 6
D. x ≥ 6
35. Factorizing `x^2 + 4x + 3` completely gives
A. (x + 4)(x + 3)
B. (X + 1)(X - 3)
C. (x - 1)(x + 4)
D. (x + 1)(x + 3)
36. Simplifying `2^-3` gives
A. -1/8
B. 1/8
C. 1/6
D. -1/6
37. The next term of the sequence -3, 1, 5, . . . is
38. The number of even nodes in the network in figure 9 is
39. The relationship that exist between sinθ, cosθ and tanθ is
A. tanθ = cosθ/sinθ
B. tanθ = sinθ/cosθ
C. tanθ = sinθ + cosθ
D. tanθ = sinθ - cosθ
40. Figure 10 is a right-angled triangle with sides n, 8cm and 6cm. THE value of n is
41. Figure 11 shows a bearing of S from R. THE bearing of R from S is
A. 026°
B. 154°
C. 206°
D. 116°
42. Given the vectors r = [-3, 6] and p = [1, -1]. EValuating r + p gives
A. [2, 5]
B. [4, 5]
C. [-2, 5]
D. [-4, 5]
43. Given the vectors OP = -4i + 3j, |OP| =
44. The direction of the vector -3i - 3j is
A. 315°
B. 135°
C. 45°
D. 225°
45. The order of the matrix (-1 0 3) is
A. 3 x 1
B. 1 x 3
C. 2 x 1
D. 2 x 3
46. The transpose of the matrix below is
47. The range of the distribution 2, 4, 2, 6, 3, 7, -1 is
48. The median of the distribution 7, 3, 1, 1, 4, 7, 0 is
49. GIven the distribution 8, 9, 6, 8, 15, 8, 11, 7. The mode is
50. The probability that Susan goes to the farm is 2/5. The probability that she does not go to the farm is
A. 3/5
B. 6/25
C. 2/5
D. 1/5
Quiz Completed
Restart Quiz















0 Reviews